Have a question about elementary mathematics content? Pedagogy? Language? Explore our library of posts answering questions teachers most frequently ask about elementary mathematics or ideas that they often want to investigate. These informational pages give insight and clarify concepts about elementary mathematics for teachers.
Do you have a question about a topic and do not see the answer below? Send a message to a member of our team!
Found 47 Results
December 3, 2019
Addition and subtraction of whole numbers can be developed together, systematically, starting with recognition of quantities and combinations of quantities, the 10-based structure of the language […]
November 20, 2019
Number tricks are fun for children. The fun, all by itself, is valuable, but is not mathematics. But understanding how the trick works is good mathematical, often algebraic, learning.
November 20, 2019
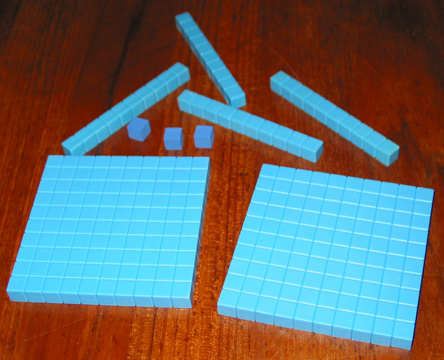
This is a school term, sometimes used in teaching math. The word has no formal mathematical definition. A model for base 10 numeration. Ten copies of […]
November 21, 2019
How to build mathematical vocabulary: Understand it well yourself (see glossary), and use it naturally (and correctly) in communication when you need it, in a rich enough context so that students can “figure out” the meaning from context, just as you might expect them to figure out the meaning of a new word that occurs in the middle of a story.
December 3, 2019
This page describes one silent teaching activity that serves, at the superficial level, to give students useful practice with multiplication facts, but that also builds deeper […]
December 3, 2019
The word dimension is related to the word measure. It is used in two ways in geometry. It is used to specify a measurement: “What are […]
November 20, 2019
The terms factor and multiple are sometimes confused with each other. Factors of 15 include 3 and 5; multiples of 15 include 30, 45, 60 (and […]
November 21, 2019
How to Play Guess My Rule games are games in which one person thinks up and gives examples of some “rule,” and the player(s) try to […]
November 21, 2019
Hundreds chart A hundreds chart in which “higher numbers” are higher, and “the 30s” are all on the same row. Unlike the number line, which is […]
January 9, 2020
Children are extraordinary linguists! They acquire half their adult vocabulary and nearly all their adult grammar by the time they are 5 years old, mostly informally […]
November 21, 2019
Area formulas Students who have the informal notion that area is the “amount of 2-D ‘stuff'” contained inside a region can invent for themselves most of […]
November 4, 2019
Length, width, height, depth Outside of the mathematics class, context usually guides our choice of vocabulary: the length of a string, the width of a doorway, […]
January 9, 2020
Informally, volume is the amount of (three-dimensional) “stuff.” The volume of a body of water is how much water there is. The volume of a sculpture […]
December 3, 2019
Mental Math exercises are lively, brief, highly focused, and daily. They are strategically selected to build students’ mental math skills quickly increase their capacity to hold […]
January 9, 2020
Standards in mathematics suitable for 21st century learners must address two kinds of knowledge: mathematical content and mathematical practice. The content standards are adjusted to reflect […]
November 21, 2019
Mathematically proficient students start by explaining to themselves the meaning of a problem and looking for entry points to its solution.